Chromatographic Theory, Part V: Chromatographic Resolution

We saw in our last column how capacity factors can be used to calculate separation factors, which are a measure of the quality of the separation between two analyte peaks. However, we found that because the separation factor ignores peak widths it can sometimes give misleading results. The solution to this problem is to calculate peak resolution, which takes both peak positions and widths into account giving a more accurate measurement of separation quality.

Separation Factors: A Review

As in all previous columns on chromatographic theory, for more details I refer you to these references (1,2).

Recall (3) that the separation factor for a pair of chromatographic peaks, which we will label as a and b, is given by Equation 1:

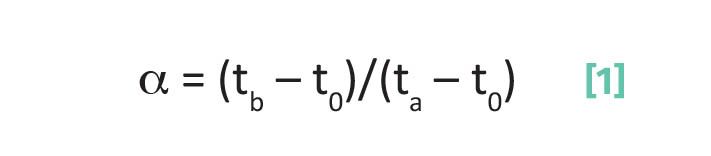
where α is the separation factor, t_b is the retention time of peak b, t_a is the retention time of peak a, t₀ is the void time, and t_b > t_a.

We also learned last time (3) that we can derive that the separation factor equals the ratio of the capacity factors for two peaks as such, seen in Equation 2:

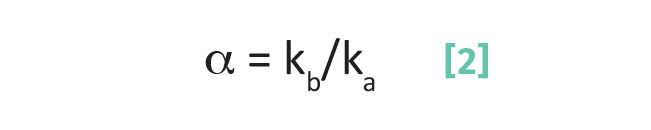
where kb is the capacity factor for peak a and ka is the capacity factor for peak b and k_b > k_a.

Figure 1 shows a chromatogram with two well separated analyte peaks marked with their capacity factors.

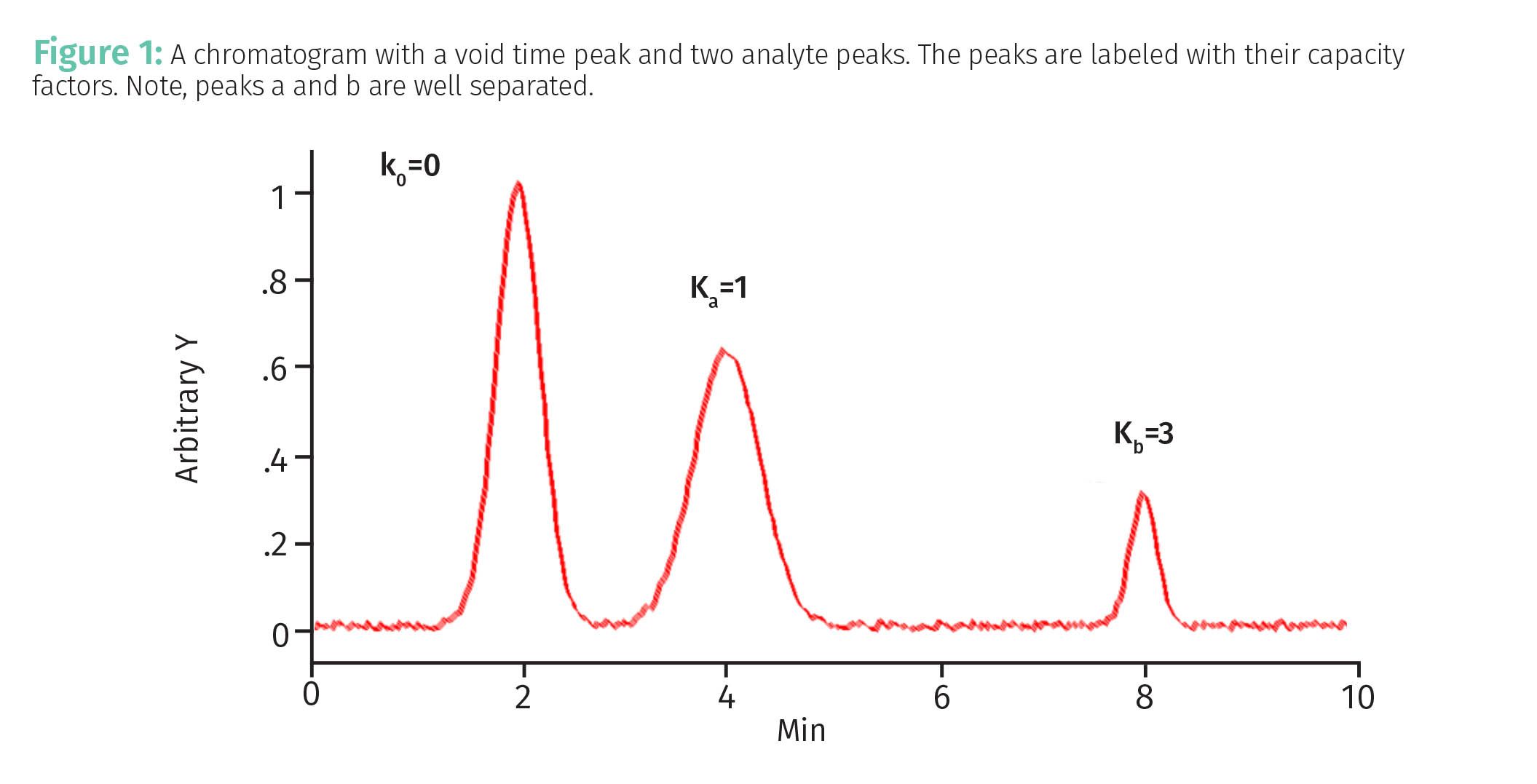
Using Equation 2 we can calculate the separation factor for peaks a and b using Equations 3 and 4:

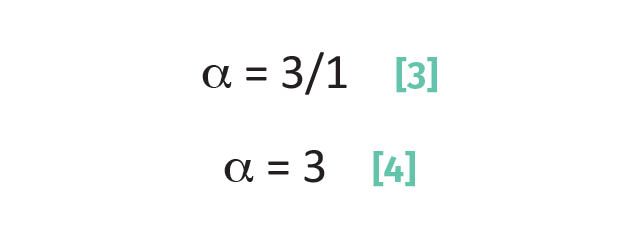
Note that analyte peaks a and b are well separated with a separation factor of 3. This is why the separation factor can be used as a measure of separation quality.

The problem with the separation factor is that it only uses elution time—the position of the top of the chromatographic peak—but ignores the fact that peaks have a width as well. We saw last time (3) that when peaks are unusually broad this does not work, as illustrated in Figure 2.

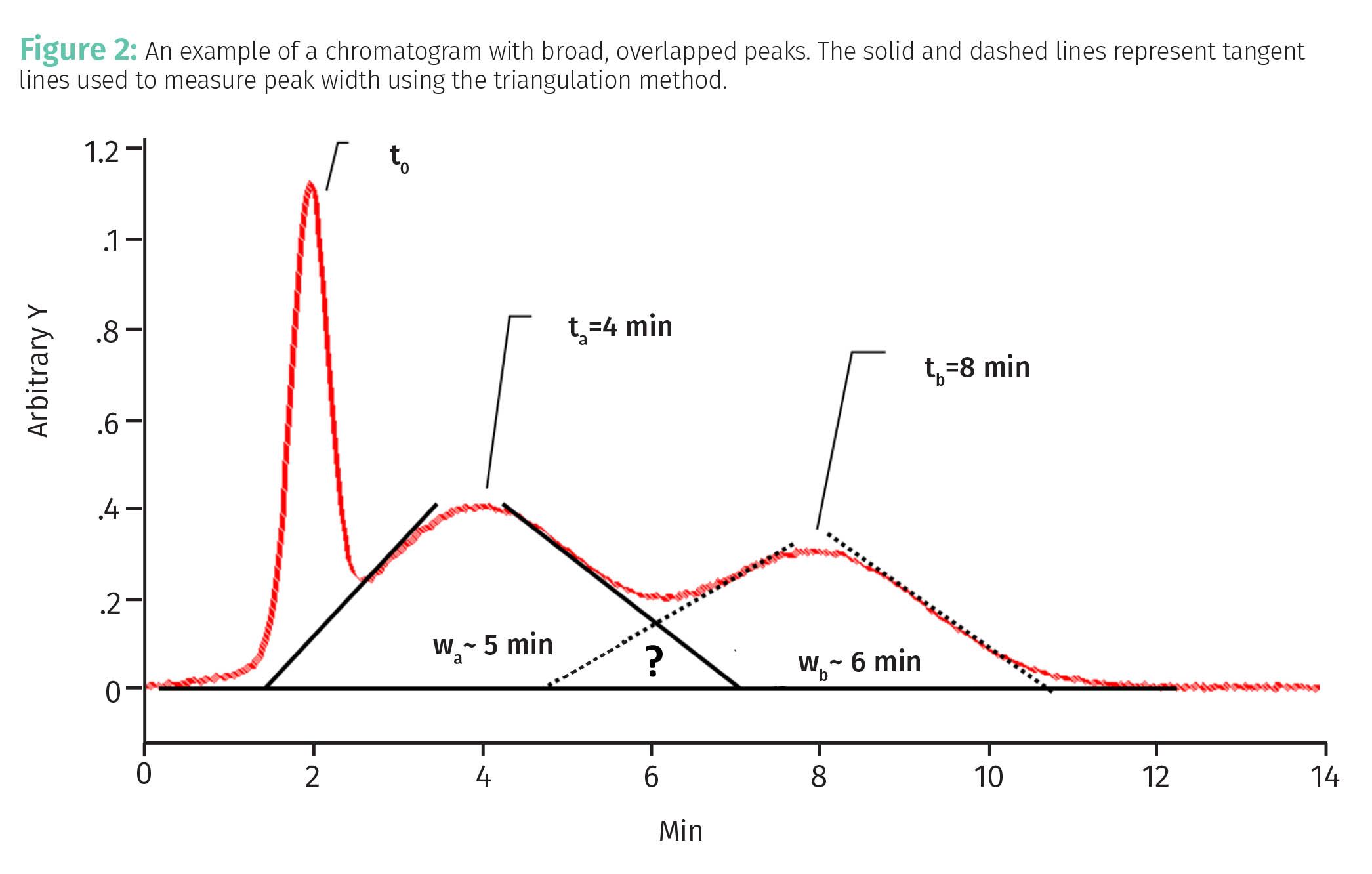
The separation factor for analyte peaks a and b for the chromatogram in Figure 2 is 3, the same as for the chromatogram in Figure 1. The problem is that the peaks in Figure 2 are poorly separated, whereas in Figure 1 they are well separated, and yet both chromatograms have the same separation factor. In Figure 2, the separation factor is not a good measure of separation quality because of large peak widths. What metric then can we use that will work for all chromatograms and all peak widths? To be able to do this we need a method of measuring chromatographic peak widths.

Measuring Chromatographic Peak Widths

Now that chromatographic systems are computerized, peak width measurements can be made easily by many different algorithms. When reporting peak widths, it is important to state how they were measured because different algorithms may produce different results. An old fashioned but useful way of measuring chromatographic peak widths is triangulation. This technique is illustrated in Figure 3.

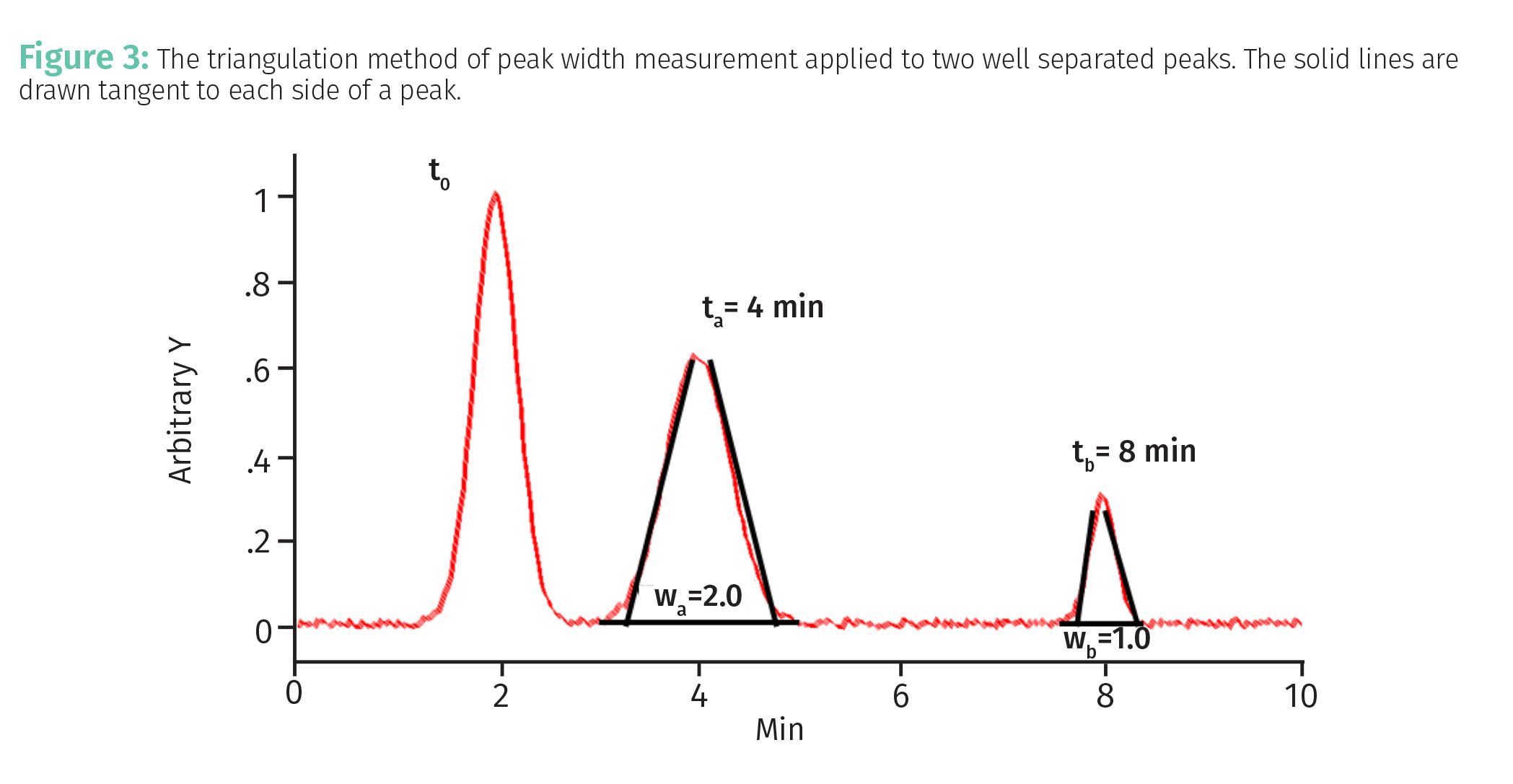
This method involves drawing tangent lines on either side of a peak as seen in Figure 3. These lines are then extended so they intersect with the baseline. The distance between the two points of intersection is the triangulated peak width. Note in Figure 3 that the width of peak a, w_a, is 2 min, and that the width of peak b, w_b, is 1 min.

The peaks in Figure 3 are well separated, making the calculation of the triangulated peak width easy. This is not the case with broad peaks as seen in Figure 2. In this case, we have two poorly separated peaks that are quite overlapped. How do we measure peak width in this case? We first draw a baseline underneath the overlapped peaks. Tangent lines are then drawn as best we can, along the sides of the portions of each peak visible, and then the tangent lines are extrapolated down to the baseline. The peak width, as above is the distance between these two intersection points. Note in Figure 2, that the width of peak a is 5 min and of peak b is 6 min. Note that these peak widths have a ~ in front of them, and there is a question mark underneath the chromatogram.

The problem with the peak width calculations made on the chromatogram in Figure 2 is that when we extrapolate the tangent lines there is a region under the chromatogram where the tangent lines for the two peaks cross, denoted by the question mark, and it is not obvious where the right edge of peak a and left edge of peak b fall. The triangulation method assumes chromatographic peaks are symmetrical and baseline separated. The purpose of the question mark is to show that this assumption may not be true.

Chromatographic Resolution

The resolution of two peaks in a chromatographic separation uses both peak position and width to measure how well peaks are separated from each other. Its form is seen in Equation 5:

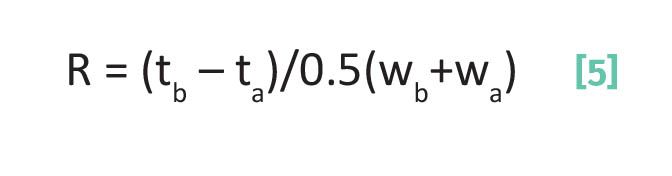
where R is the resolution, t_b is the elution time for peak b, t_a is the elution time for peak a, w_a is the width of peak a, and w_b is the width of peak b, with t_b > t_a.

Note in the numerator of Equation 5, that the elution times for two chromatographic peaks are subtracted from each other. The stipulation that tb be greater than ta exists so that the numerator, and hence R, have a positive value. Note that the denominator in Equation 5 contains the width of peaks a and b. Finally, we have a measure of separation quality that includes width. Remember that the widths of peaks a and b are added together and divided by 2; that is, the denominator is the average of the two peak widths. Since the average of the peak widths is in the denominator, as this average goes up R goes down. That is, broader peaks give a lower resolution value than narrower peaks.

We saw above that the separation factor fails as a measure of separation quality for broad peaks, and the separation factors for peaks a and b in Figures 1 and 2 were the same, even though these separations are clearly of different quality. How then does our new metric, the resolution, handle this situation? For the well separated peaks in Figure 3 the resolution is seen in Equations 6 and 7:

For the poorly separated peaks in Figure 2 the resolution is seen in Equations 8 and 9:

Ha! We finally have it, the resolution, a metric that properly measures separation quality in the presence of broad peaks by using peak width in its calculation. Note that the well separated peaks in Figure 3 have an R value well above 1, while the poorly separated peaks in Figure 2 have an R value below 1. A resolution of 1 means two peaks are 3% overlapped, and an R of 1.5 or greater means two peaks are baseline resolved, as is the case in Figure 3. Remember that chromatography is used primarily as a quantitative technique, and that peak heights and areas are used to determine concentrations of analytes in samples. For this quantitation to be at its most accurate, baseline resolution of all peaks in a chromatogram is necessary. Thus, when developing methods our goal is to make sure that R for all pairs of peaks is at or above 1.5. The question then becomes what experimental variables can be adjusted, in what direction, to achieve R values of 1.5 or greater? You will have to wait for future columns for the answer.

This column has been devoted to including peak width in our separation quality calculations. But why are some chromatographic peaks narrow and others wide? In other words, what determines chromatographic peak width? You will have to read the next column to find out.

Conclusions

We reviewed the concept of separation factors, and found them wanting because they ignore peak widths, leading to situations where well separated and poorly separated pairs of peaks have the same value of α. The solution to this problem necessitates developing a method for measuring peak width. A tried-and-true method that was illustrated was triangulation. We then introduced the concept of chromatographic resolution for a pair of peaks, and since this quantity includes peak widths, we found it accurately measures peak separation even in the presence of broad peaks.

References

1. Skoog, D.A., West, D.M., and Holler, E.J., Analytical Chemistry: An Introduction, Saunders College Publishing, 1994, 6th Edition.
2. Robinson, J.W., Undergraduate Instrumental Analysis, Marcel Dekker, 1995, 5th Edition.
3. Smith, B., Chromatographic Theory, Part IV: Measuring Separation Quality with Capacity and Separation Factors, Cannabis Science and Technology, 2023, 6(3), 8-11.

About the Columnist

Brian C. Smith, PhD, is Founder, CEO, and Chief Technical Officer of Big Sur Scientific. He is the inventor of the BSS series of patented mid-infrared based cannabis analyzers. Dr. Smith has done pioneering research and published numerous peer-reviewed papers on the application of mid-infrared spectroscopy to cannabis analysis, and sits on the editorial board of Cannabis Science and Technology. He has worked as a laboratory director for a cannabis extractor, as an analytical chemist for Waters Associates and PerkinElmer, and as an analytical instrument salesperson. He has more than 30 years of experience in chemical analysis and has written three books on the subject. Dr. Smith earned his PhD on physical chemistry from Dartmouth College. Direct correspondence to: brian@bigsurscientific.com.

How to Cite this Article:

Smith, B., Chromatographic Theory, Part V: Chromatographic Resolution, Cannabis Science and Technology, 2023, 6(4), 8-11.